Lesson Modules
Teaching Tips:
Show the video to the class and have students answer the poll question. Encourage students to use only on word in the answer box. If there is a space in their answer, the system won't recognize the anwer and exclude from the word cloud results.
Share the poll result and have a quick class discussion (less than 5 minutes). Lead the discussion toward to importance information to solve the challenge. In the next module, students will explore strategies.
Watch the video and answer the following question.
Teaching Tips:
- Anticipate some possible strategies student might use:
- draw a line segment between the two points.
- use coordinates
- use the right triangle and use the sine/cosine
- use a protractor to find the angles
- use a ruler to measure the distance directly
- Choose 2-3 different strategies you want to discuss with your class.
- While the students are working in groups monitor who is working on what strategy.
- Make a sequence of the strategies you want the students to present.
- Have a discussion on the similarities and the differences between the strategies and have students think about the cons. and pros.on each strategy.
- For example, using a ruler can be easy and visual, but it doesn't give the precise measure. Remember the goal of this lesson is to use the Pythagorean theorem to conclude the distance formula. We recommend beginning the presentation with a concrete strategy such as using a ruler and lead to the strategy of using the distance formula. Make sure each strategy is connected, for example, using the right triangle can be after the line segment.
Use the interactive tool below.
Direction:
- Click on the box next to "Goal Post" and see where the goalposts locate.
- Repeat clicking on the box and see where is the item is located.
- Remember the distance formula is made by the Pythagorean Theorem!
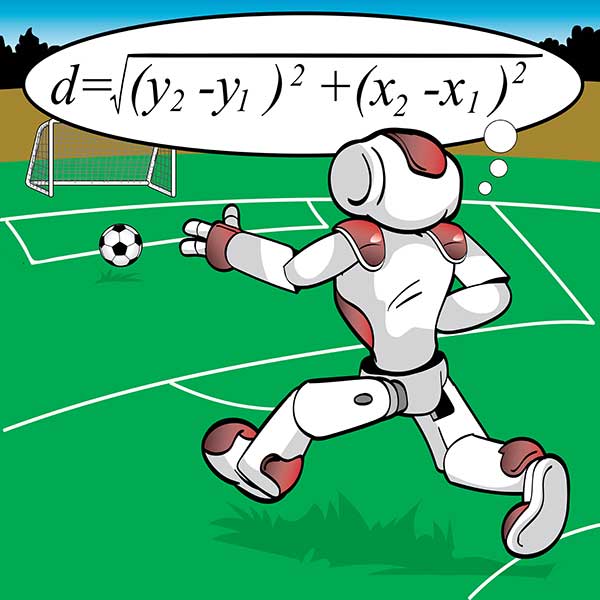
Your Challenge:
Find the distance between the ball position and one of the goal post. You can use any strategies to figure out the distance. Free yourself! Be creative!
Teaching Tips:
Have students play with the simulation before connecting the physical robot.
Call a few students or have each group connect the physical robot to try their answers. If the answer is correct, the robot approaches the ball and kicks to score the goal. If the answer is incorrect, the robot says "I am sorry the perimeter is actually smaller/bigger than that. I cannot score the goal"
You can facilitate the game environment as a team competition for this activity.
{{simulation
Teaching Tips:
Have students answer the questions individually in a quiet environment.
The results will appear in the class view. Share with the students to see how the class did in this lesson.
You've learned how to find the distance between two points on the coordinate plane today. Think of how you did in this lesson and refelct on how much you understand. This is not a test. Be honest on evaluating your understanding. Your teacher will use the data to plan better the next lesson.
- Not at all
- Kind of got it
- Understand
- Pretty good
- Totally got it